User:Valerie

Sandbox

                                  ==  $%$ v@13R!3 $%$  ==

FÄTT KNUSPERKNUSPER WE_LOVE_BODEV

unser Bild

das ist unser plakat für das kambotscha projekt

y

x(t)=x₀ + vt

x(t)= [math]x_0[/math] + v*t

[math]\Delta x[/math] = v* [math]\Delta t[/math]

v= [math]\frac {x}{t} [/math]

v= [math]\frac {x_1-x_0}{\Delta t}[/math]

[math]x_1[/math](t)= [math]x_2[/math](t)

[math]x_1+v_1*t = x_2+v_2*t[/math]

[math]x_1-x_2 = (v_2-v_1)*t[/math]

[math]t = \frac {x_1-x_2}{v_2-v_1}[/math] = [math]\frac {173m-25m}{28km/h-11km/h}[/math]

VERBESSERUNG DER 1. EXTEMPORALE

a)

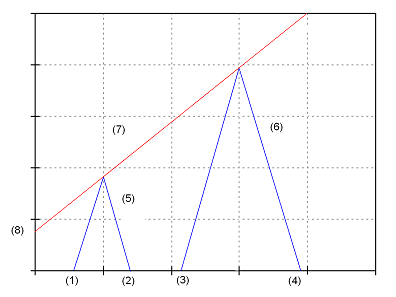Rechtswert: Zeit t , Hochwert: Ortskoordinate x

b) x₁ = v * [math]\frac {\Delta t} {2} [/math]

  [math]\Delta t[/math] = t2 - t1
  = 0.30s

sandbox

Exercise 2.7: From zero to hundred

The test run of a new motorbike gave a rate growth from 0 to 100 km/h in 3,87 s. WORK OUT THE middle speedup and the way it put back at this speedup and this time!

Solution

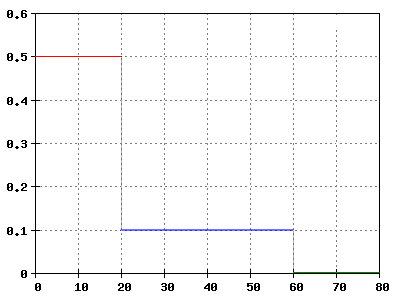
Exercise 2.8: Train ride

Easting: time t in s

Northing: speedup a in m/s^2

A train starts moving. The diagram shows the dependence of its middle speedup of time.

a) Work out the rate growth from the train after 20 s, 60 s and 80 s and draw the t-v-diagram b) Work out with the help of the t-v-diagram the way it put back for the same point of time and draw a t-x-diagram

Solution

Exercise 2.9: Bullet

The ball of a gun should constant speed up in its barrel.

a) After what time the ball leaves the barrel b) How big is the speedup if the 18 cm long barrel will be left with 760 m/s.

Solution

Exercice 2.10: Cavalier’s start?

At the start of a car you grab the following measured values:

Zeit t in s	Ort x in m
0	0
1	0,5
2	2
3	4,5
4	8
4,2	8,8
5	12
6	16
7	20

a) With which way-time-functions the movements leave themselves in time sections to 0 < t < 4 s and 4 s < t < 7 s describe? b) Intend acceleration and the momentary speed for each point of the chart! Draw the (t) diagram! Determine from it with the help of the surface the place x(6,5 s) to t = 6.5 s!

Solution

Aufgabe 3.7: Gehalten !

a)

Lösung: v² = 2ax

--> a = v²/2x

a = 3836 m/s²

F = m * a

F = 0,50 kg * 3836 m/s²

F = 1918 N

Aufgabe 4.3: Spann die Feder

geg.: F = 50 N s = 80 cm = 0,8 m

a) ges.: W_sp

Lösung: F = D*s => D = F/s = 50 N/0,8 m = 62,5 N/m

W_sp = 1/2 D*s = 1/2 * 62,5 N/m * (0,8)² = 20 J

Schleuderball mit Tücken

geg:

• m = 0,80 kg
• r = 50 cm
• v = 3,4 m/s

ges:Fz im höchsten und tiefsten punkt?

   Frequenz bei der die schnur reißt wenn Reißfestigkeit 50N beträgt?
   Mit welcher Geschwindigkeit fliegt der Ball waagrecht weg?

Lösung:

[math]u = 2r\pi = 3,14 m \frac{}{}[/math]

[math]T = \frac{u}{v}[/math]

[math]T = \frac{3,14 m}{3,4 m}[/math]

[math]T = 0,924 s \frac{}{}[/math]

[math]\omega = \frac{2\pi}{T}[/math]

[math]F_Z = m\omega^2r \frac{}{}[/math]

[math]F_Z = 0,80 kg * (\frac{2\pi}{0,924s})^2 * 0,50m[/math]

[math]F_Z = 18,49 N \frac{}{}[/math]

[math]g = 9,81 \frac{N}{kg}[/math]

[math]F_Z = g * 0,8 kg \frac{}{}[/math]

[math]F_Z = 9,81 * \frac{N}{kg} * 0,8 kg[/math]

[math]F_Z = 7,84 N \frac{}{}[/math]

[math]F_{Z.oben} = F_Z - F_{Z.grav} \frac{}{}[/math]

[math]F_{Z.oben} = 10,65 N \frac{}{}[/math]

[math]F_{Z.unten} = F_Z + F_{Z.grav} \frac{}{}[/math]

[math]F_{Z.unten} = 26,35 N \frac{}{}[/math]

Ausflug auf den Reiterhof:

Pferdchen Detlef D! Soost läuft an einer Leine von 8m einen im Kreis in 45s.

a) Berechne die Winkelgeschwindigkeit und die normale Geschwindigkeit.

b) Berechne die Freuquenz die Detlef D! Soost in 3 Runden zurück legt.